Research
Block Copolymers and Nano-Imprint Lithography
Producing a surface with an ultra-dense array of addressable nanoscopic elements that is perfectly ordered over macroscopic length scales is a formidable challenge. The self-assembly of di-block copolymers (BCP), two chemically dissimilar polymers joined together, is emerging as a promising route to generate templates and scaffolds for the fabrication of nanostructured materials and offers a potential solution to this challenge. Furthermore, it has been considered as a legitimate next-generation microelectronics lithography technique for insertion at the sub-22 nm technology nodes.
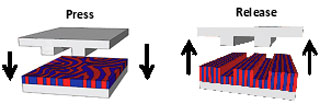
Nano-imprint lithography is a new method for macroscopic alignment of BCP on molecular scale. It can be used as a tool for locally controlling the self-assembly of BCP and determining the precise position of the phase-separated domains. The idea behind the NIL technique is to press a hard and structured mold into the BCP film at temperatures higher than the glass temperature of the polymer, inducing the preferred nano-structures in the BCP film. The method is high throughput and low cost, and can achieve sub-10 nm resolution.
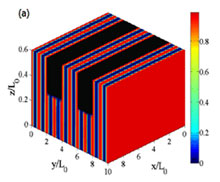
Self-consistent-field theory (SCFT) has been used to explore how lamellar phase of symmetric BCP are aligned and oriented in a Nano-Imprint Lithography setup. We have chosen a three dimensional system of size ( is the natural periodicity of BCP lamellae), and performed a gradual temperature quench from a temperature above the order-disorder temperature into the strong segregation region. With the simulated Nano-Imprint mold, we find a perfect perpendicular lamellar structure, shown in (a).
As a further check, we compared the Nano-Imprint setup with a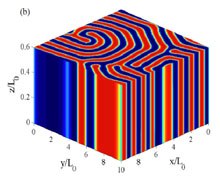 
BCP film confined between two neutral and flat surfaces. As can be seen in (b), in the latter case the film contains many in-plane defects when the same gradual temperature quench process is repeated. Without the Nano-Imprint mold, it is possible to obtain perpendicular lamellae but only with many in-plane defects that cannot be annealed away. On the other hand, with the Nano-Imprint mold, wetting of the vertical groove wall induces perfect perpendicular ordering with minimal amount of defects, over large lateral distances.
For further reading:
- "Block Copolymers at Nano-Patterned Surfaces", X. K. Man, D. Andelman, and H. Orland; Macromolecules 43, 7261 (2010).
- "Nanoscale Organization of Block Copolymers at Surfaces: Comparison of Theory and Experiments", X. K. Man, D. Andelman, H. Orland, P. Thebault, P.-H Liu, P. Guenoun J. Daillant, and S. Landis; Marcomolecules 44, 2206 (2011).
Ion-Specific Effects
Franz Hofmeister observed ion-specific effects already in the late 1800's and showed that certain monovalent ions (such as fluoride and chloride) are more effective at precipitating proteins ("salting out") than others, such as bromide and iodide. These effects are found to influence the interactions of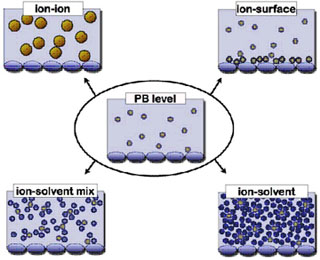
surfactant micelles, lipid-bilayer membranes, proteins, DNA molecules and more. Understanding these effects theoretically is of major importance.
Our strategy to tackle the problem is to use models on the mean-field level and beyond, with which an intuitive picture of the various effects emerges. Several such models have been accounted by us, where we extended the regular Poisson-Boltzmann theory to include other electrostatic and non-electrostatic interactions in the system free energy. Among these are steric, solvation, and dipolar interactions.
For further reading:- "Dipolar Poisson-Boltzmann Equation: Ions and Dipoles Close to Charge Interfaces”, A. Abrashkin, D. Andelman, and H. Orland, Phys. Rev. Lett. 99, 0077801 (2007).
- "Beyond Standard Poisson-Boltzmann Theory: Ion-Specific Interactions in Aqueous Solutions”, D. Ben-Yaakov, D. Andelman, D. Harries, and R. Podgornik, J. Phys.: Condens. Matter 21, 424106 (2009).
- "Dielectric Decrement as a Source of Ion-Specific Effects”, D. Ben-Yaakov, D. Andelman, and R. Podgornik, J. Chem. Phys. 134, 074705 (2011).
- "Ion-Specific Hydration Effects: Extending the Poisson-Boltzmann Theory, D. Ben-Yaakov, D. Andelman, R. Podgornik, and D. Harries”, http://arxiv.org/abs/1103.4590
Ion-Induced Surface and Solvent Transitions
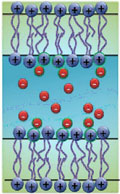It is well known that the presence of ions lead to modification of other species such as solvent and surface groups. For example, the force between two charged objects (such as lipid membranes, DNA strands and charged colloids) is strongly influenced by the distribution of the ions in between these two objects. Furthermore, the structural properties of these systems can also be affected by the presence of the ions. We are interested in systems where the interactions of the ions with the other species, e.g., lipids in the membrane, or solvent molecules, lead to modifications of the structural properties.
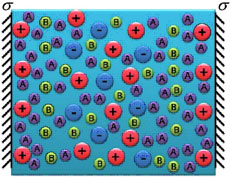In particular, we explained a lamellar-lamellar phase transition that was observed experimentally in terms of electrostatics and charge regulation mechanism. Interestingly, the charge regulation mechanism is ion-specific. In another study, we suggested a model to explain the inter-lamellar force in systems where the solvent is composed of two different liquids. Here the rearrangement of the solvent composition due to the presence of the ions, lead to a reduction of the force between two planar charged plates.
- “Ion Induced Lamellar-Lamellar Phase Transition in Charged Surfactant Systems”, D. Harries, R. Podgornik, V.A. Parsegian, E. Mar-Or, and D. Andelman; J. Chem. Phys. 124, 224702 (2006).
- “Ions in Mixed Dielectric Solvents: Density Profiles and Osmotic Pressure between Charged Interfaces”, D. Ben-Yaakov, D. Andelman, D. Harries, and R. Podgornik; J. Phys. Chem. B 113, 6001 (2009).
Coupled Modulated Bilayers
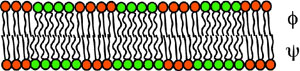
Our motivation is related to recent experiments by Collins and Keller who investigated Montal–Mueller planar bilayer membranes composed of lipids and cholesterol. With this technique, a bilayer is constructed by separately preparing two independent monolayers and then combining them into one joint bilayer across a hole at the air/water interface. The experiments specifically addressed the question of liquid domains in the two leaflets, and the mutual influence of the monolayers in terms of their domain phases. In the experiment, asymmetric bilayers were prepared in such a way that one leaflet’s composition would phase-separate in a symmetric bilayer and the other’s would not. In some cases, one leaflet may induce phase separation in the other leaflet, whereas in other cases, the second leaflet suppresses domain formation in the original leaflet. These results imply that two-leaflet coupling is an important ingredient in determining the bilayer phase state. Motivated by these experiments, the coupled bilayer system was investigated theoretically. The coupling mechanism arises through interactions between lipid tails across the bilayer midplane, and the phase behavior of such a bilayer membrane was computed using either regular solution theory[14] or Landau theory.[15] The theoretical results are in accord with several of the experimental observations. It should be noted that all previous models dealt with the coupling between two macro-phase-separated leaflets, while it is also of interest to investigate the coupling between two micro-phase-separated (modulated) leaflets. Furthermore, one might also consider the interplay between a macro- and a micro-phase separation.
Herein, we suggest a model describing the coupling between two modulated systems, and, in particular, we analyse the influence of this coupling on the phase behavior of two coupled 2D monolayers. When the two monolayers have the same preferred periodicity of modulation, we obtain mean field phase diagrams that exhibit various combinations of micro-phase-separated structures. In some cases, the periodic structure in one of the monolayers induces a modulation in the other monolayer. Interesting situations take place when
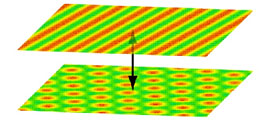We propose a model addressing the coupling mechanism between two spatially modulated monolayers. We obtain the mean-field phase diagrams of coupled bilayers when the two monolayers have the same preferred modulation wavelength. Various combinations of the monolayer modulated phases are obtained and their relative stability is calculated. Due to the coupling, a spatial modulation in one of the monolayers induces a similar periodic structure in the second one. We have also performed numerical simulations for the case when the two monolayers have different modulation wavelengths. Complex patterns may arise from the frustration between the two incommensurate but annealed structures.
For further reading:
- “Coupled Modulated Bilayers: A Phenomenological Model”, Y. Hirose, S. Komura, and D. Andelman; ChemPhysChem 10, 2839 (2009).