
Answer to the Question 02/02
MAXIMAL GRAVITY
The question was:
Consider a celestial body (planet) of mass M
and volume V consisting of material of uniform
density M/V. If the body is spherical, then its
radius is R=(3V/4{pi})1/3, and the free-fall
acceleration on its surface is
g=GM/R2=GM(4{pi}/3V)2/3,
where G is the gravitational constant. Consider all
possible shapes of the body. What is the largest g,
that can be achieved at one point on a surface, and what is
the shape of the body for which such acceleration is achieved?
(5/2002) The problem has been solved (9/2/2002)
by Eva Sandlokk (e-mail
eva.sandlokk@prodigy.net),
(18/2/2002) by Kirk T McDonald from Henry Laboratories,
Princeton, NJ (e-mail
mcdonald@puphed.princeton.edu)
- see his solution in PDF format, as well as
by Mattew Horton (1/4/2002) (e-mail
matthew.horton@ssmb.com),
and (2/4/2002) Daniel Gulotta from Illinois Mathematics and Science Academy
(e-mail
dgulotta@imsa.edu).
While direct analytical solution of the problem is possible, a very interesting
approach was suggested (2/4/2002) by Horst Schneider from
the Physics Department
in Martin-Luther-Universitat Halle-Wittenberg in Germany (e-mail
schneider@physik.uni-halle.de); the approach begins with numerical optimization, and eventually
arrives to an analytical solution - see files 1
and 2.
It was also partially
solved (26/3/2002) by Jared Daniel Kaplan from Stanford
(e-mail jaredk@Stanford.edu).
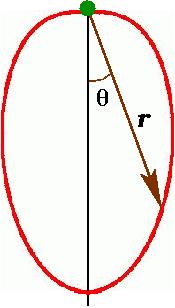
The answer: The optimal body has axial symmetry and has the shape depicted
at the picture below. The green dot denotes the point of maximal free-fall
acceleration. The body can be described
by relating distance r from the point at which the maximal
acceleration is found as a function of the angle {theta} from
the axis of symmetry (vertical black line).
r2=R2cos({theta}).
The acceleration at the green point will be
g=(4/5)(15/4)1/3{pi}2/3M/V2/3.
This is only 2.6% larger than the gravity on the surface of a spherical
planet.
The solution:
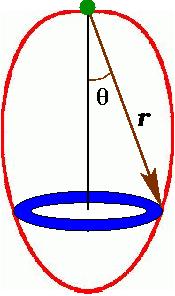
Assume for a moment that we already know that the body should have
axial symmetry. Consider a narrow ring of matter lying on the surface
of the body. (Center of the ring coincides with the axis of symmetry and
its plane is perpendicular to that axis. It is depicted on the figure below.)
If the mass of the ring is
dM and the distance between the points of the ring and the
"green point" is r, then the contribution of the ring to
free-fall acceleration will be G*{dM}cos({theta})/r2.
If we remove this ring of material from the surface and place it
somewhere else on the surface, it will have different r and
different angle {theta}. Since the shape is optimal, it is
"stationary", i.e. infinitesimal changes should not change the
acceleration. This will happen only if cos({theta})/r2
is constant. Consequently,
r2=R2cos({theta}). The constant
R denotes the maximal diameter of the body.
Once the shape of the body is given, we can easily find (by an elementary
integration*) that its volume
V=(4/15){pi}R3, while the acceleration at point
g is given** by the expression which has been presented above.
Using similar argument you can now show that the body must have axial
symmetry. Imagine that is not so, i.e. the ring which was described above
is not complete - then you can easily see that the free-fall acceleration can
be increased by relocating some of the surface material.
For a more detailed solution see PDF format.
Comments:
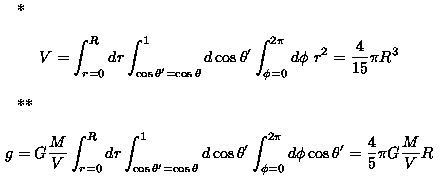
 Back to "front page"
Back to "front page"



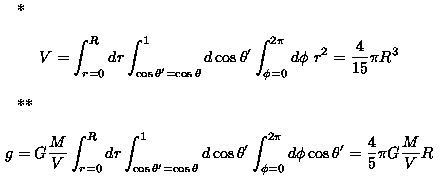
 Back to "front page"
Back to "front page"